Principle of Pattern Multiplication

1. The principle of pattern multiplication states that “the radiation pattern of an array is the product of the pattern of the individual antenna with the array pattern of isotropic point sources each located at the phase centre of the individual source.”

2. The array pattern is a function of the location of the antennas in the array and their relative complex excitation amplitudes.

3. The phase centre of the array is the reference point for total phase pattern.

4. Advantage: It helps to sketch the radiation pattern of array antennas rapidly from the simple product of element pattern and array pattern.

5. Disadvantage: This principle is only applicable for arrays containing identical elements.

6. The principle of pattern multiplication is true for any number of similar sources.

7. Total phase pattern is the addition of the phase pattern of the individual sources and that of the array of isotropic point sources.

The resultant pattern of an array of non-isotropic identical radiators is given by

E = [f (θ, ϕ) × F (θ, ϕ)] × [f_P (θ, ϕ) + F_P (θ, ϕ)]

where,

f (θ, ϕ) = element field pattern

f_P (θ, ϕ) = element phase pattern

F (θ, ϕ) = array factor of isotropic elements

F_P (θ, ϕ) = phase pattern of the array of isotropic elements.

The angles θ and ϕ respectively represent the polar and azimuth angles.

EXAMPLE 1: Consider an array of four elements (isotropic or non-directional):

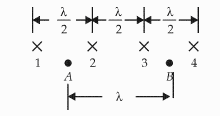
• Elements are spaced at λ/2.
• Elements 1 and 2 are considered as one unit, 3 and 4 are considered as one unit.
• Since the elements are identical, both the units have the same radiation pattern.
• The unit pattern is the pattern of two elements spaced at λ/2, given below:

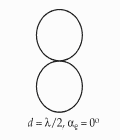
• The units represented by A and B are separated by λ. These two units are considered to be one unit whose radiation pattern is shown below:

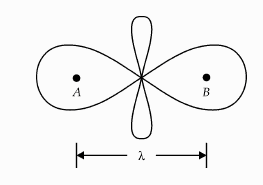
• Therefore, the resultant pattern is given by the product of unit pattern (λ/2 spacing) of 1 and 2 elements or 3 and 4 elements and a group pattern (λ spacing) of A and B.

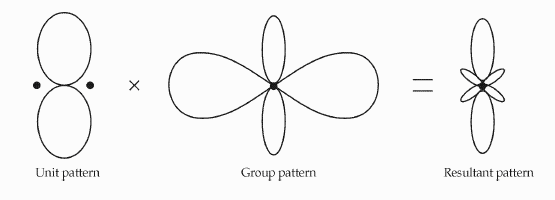
EXAMPLE 2: Consider an array of eight elements spaced at λ/2 (isotropic or non-directional):

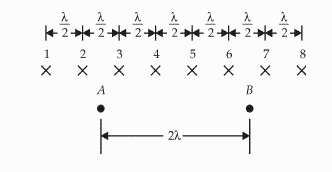
• Centre of the first four elements and last four elements are marked as A and B.
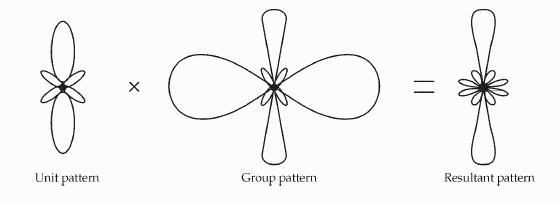
• The unit pattern is the pattern of four elements.
• The group pattern is the pattern of two elements spaced at 2λ.
• The resultant pattern is again the product of unit pattern and group pattern.